複素数平面 での複素数の絶対値 r , 偏角 φ 。数学 において、複素数 の偏角 (へんかく、英 : argument of complex )とは、複素数平面 上で複素数が表す点の動径 が表す一般角 のことである。複素数 z の偏角は記号で arg z で表す。偏角はラジアン で表す。
複素数を極形式表示することで、絶対値 と偏角が得られる。これにより、複素数の乗除が簡明に行うことができる。
複素数に対する偏角は、2π の任意の整数倍を足す分だけ表し方がある。つまり、多価関数 である。そこで表示を一意にするには、主値 区間 (−π , π ] などに制限する。
2π の任意の整数倍の差を除いて次の等式が成り立つ:
arg zw ≡ arg z + arg w arg z / w z − arg w (何れも mod 2π ) 偏角 φ の2つの選び方 複素数 z = x + yi 偏角 は、arg z と書かれ、正の実 軸 から動径 Oz までの角度を反時計回りに測った角度である。弧度法 で表示する。時計回りに測ると負になる。
複素数に対する偏角の表示を一意にするために、主値 区間 (−π , π ] に制限する。[0, 2π ) にすることもある。
主値を (−π , π ] にすると、逆正接関数 tan−1 を用いて次のように表せる:
arg z = { tan − 1 y x ( x > 0 ) tan − 1 y x + π ( x < 0 ∧ y ≧ 0 ) tan − 1 y x − π ( x < 0 ∧ y < 0 ) π 2 ( x = 0 ∧ y > 0 ) − π 2 ( x = 0 ∧ y < 0 ) indeterminate ( x = y = 0 ) {\displaystyle \arg z={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}&(x>0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}+\pi &(x<0\,\land \,y\geqq 0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}-\pi &(x<0\,\land \,y<0)\\[0.1em]{\dfrac {\pi }{2}}&(x=0\,\land \,y>0)\\[0.1em]-{\dfrac {\pi }{2}}&(x=0\,\land \,y<0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}} 上記の式には条件分岐が多数あるが、符号関数 sgn やヘヴィサイドの階段関数 H (x )
arg z = { tan − 1 y x + 1 − sgn x 2 ( 1 + sgn y − | sgn y | ) π ( x ≠ 0 ) ( sgn y ) π 2 ( x = 0 ∧ y ≠ 0 ) indeterminate ( x = y = 0 ) = { tan − 1 y x + { 1 − H ( x ) } { 2 H 1 ( y ) − 1 } π ( x ≠ 0 ) ( sgn y ) π 2 ( x = 0 ∧ y ≠ 0 ) indeterminate ( x = y = 0 ) {\displaystyle {\begin{aligned}\arg z&={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}+{\dfrac {1-\operatorname {sgn} x}{2}}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\pi &(x\neq 0)\\[0.1em](\operatorname {sgn} y){\dfrac {\pi }{2}}&(x=0\,\land \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}+\{1-H(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0)\\[0.1em](\operatorname {sgn} y){\dfrac {\pi }{2}}&(x=0\,\land \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}} 0 × (0 除算を含む式) = 0 と形式的に考えることで、更にまとめることもできる:
arg z = { | sgn x | tan − 1 y x + 1 − sgn x 2 ( 1 + sgn y − | sgn y | ) π ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) = { | sgn x | tan − 1 y x + { 1 − H 1 / 2 ( x ) } { 2 H 1 ( y ) − 1 } π ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) {\displaystyle {\begin{aligned}\arg z&={\begin{cases}|\operatorname {sgn} x|\tan ^{-1}{\dfrac {y}{x}}+{\dfrac {1-\operatorname {sgn} x}{2}}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}|\operatorname {sgn} x|\tan ^{-1}{\dfrac {y}{x}}+\{1-H_{1/2}(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}} あるいは、逆余弦関数 cos−1 や逆正弦関数 sin−1 を用いて次のように表すこともできる:
arg z = { ( 1 + sgn y − | sgn y | ) cos − 1 x | z | ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) = { { 2 H 1 ( y ) − 1 } cos − 1 x | z | ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) {\displaystyle {\begin{aligned}\arg z&={\begin{cases}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\cos ^{-1}{\dfrac {x}{|z|}}&(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\{2H_{1}(y)-1\}\cos ^{-1}{\dfrac {x}{|z|}}&(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}} arg z = { ( 1 + sgn x − | sgn x | ) sin − 1 y | z | + | sgn x | − sgn x 2 ( 1 + sgn y − | sgn y | ) π ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) = { { 2 H 1 ( x ) − 1 } sin − 1 y | z | + { 1 − H 1 ( x ) } { 2 H 1 ( y ) − 1 } π ( x ≠ 0 ∨ y ≠ 0 ) indeterminate ( x = y = 0 ) {\displaystyle {\begin{aligned}\arg z&={\begin{cases}(1+\operatorname {sgn} x-|\operatorname {sgn} x|)\sin ^{-1}{\dfrac {y}{|z|}}+{\dfrac {|\operatorname {sgn} x|-\operatorname {sgn} x}{2}}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\{2H_{1}(x)-1\}\sin ^{-1}{\dfrac {y}{|z|}}+\{1-H_{1}(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}} ここで、|z | は複素数の絶対値 で、|z | = √ x 2 + y 2 である。
主値を [0, 2π ) にするには、上記の定義で、負となる偏角の値に対しては 2π を加えることにすればよい。
偏角を「位相 」[ 1] [ 2]
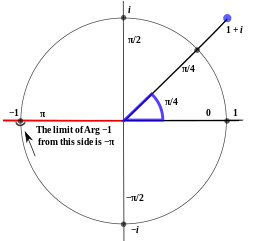
| z | cos ( arg z ) = Re z {\displaystyle |z|\cos(\arg z)=\operatorname {Re} z} | z | sin ( arg z ) = Im z {\displaystyle |z|\sin(\arg z)=\operatorname {Im} z} arg z ¯ = − arg z {\displaystyle \arg {\bar {z}}=-\arg z} arg 0 {\displaystyle \arg 0} 1 + i (青点)の主値 Arg は π / 4 主値 (−π , π ] における偏角の値を、記号で Arg z (最初の文字を大文字)で表すことがある。表記には揺れがあり、arg と Arg が文献によって逆になることもあることに注意。
arg z = { Arg z + 2 π n ∣ n ∈ Z } {\displaystyle \arg z=\{\operatorname {Arg} z+2\pi n\mid n\in \mathbb {Z} \}} Arg z = { arg z − 2 π n ∣ n ∈ Z ∧ ( − π < Arg z ≦ π ) } {\displaystyle \operatorname {Arg} z=\{\arg z-2\pi n\mid n\in \mathbb {Z} \,\land \,(-\pi <\operatorname {Arg} z\leqq \pi )\}} 複素数 z = x + yi 逆正接関数 arctan y / x で表せる。
x > 0−π / 2 z < π / 2
Arg z = tan−1 y / x が成り立つが、x > 0x < 0y > 0y < 0
Arg ( x + i y ) = { tan − 1 y x ( x > 0 ) tan − 1 y x + π ( x < 0 ∧ y ≧ 0 ) tan − 1 y x − π ( x < 0 ∧ y < 0 ) π 2 ( x = 0 ∧ y > 0 ) − π 2 ( x = 0 ∧ y < 0 ) indeterminate ( x = y = 0 ) {\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}&(x>0)\\[0.2em]\tan ^{-1}{\dfrac {y}{x}}+\pi &(x<0\,\land \,y\geqq 0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}-\pi &(x<0\,\land \,y<0)\\[0.1em]{\dfrac {\pi }{2}}&(x=0\,\land \,y>0)\\[0.1em]-{\dfrac {\pi }{2}}&(x=0\,\land \,y<0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}} 上半平面、下半平面ごとに表示することもできる:
Arg ( x + i y ) = { π 2 − tan − 1 x y ( y > 0 ) − π 2 − tan − 1 x y ( y < 0 ) 0 ( x > 0 ∧ y = 0 ) π ( x < 0 ∧ y = 0 ) indeterminate ( x = y = 0 ) {\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}{\dfrac {\pi }{2}}-\tan ^{-1}{\dfrac {x}{y}}&(y>0)\\[0.1em]-{\dfrac {\pi }{2}}-\tan ^{-1}{\dfrac {x}{y}}&(y<0)\\[0.1em]0&(x>0\,\land \,y=0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}} Arg の主値を区間 [0, 2π ) とする変種では、値が負のときに値に 2π を足すことで得られる。
正接の半角公式 tan θ / 2 sin θ / 1 + cos θ を用いると、1つの計算式で表せる:
Arg ( x + i y ) = { 2 tan − 1 y x 2 + y 2 + x ( x > 0 ∨ y ≠ 0 ) π ( x < 0 ∧ y = 0 ) indeterminate ( x = y = 0 ) {\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}2\tan ^{-1}{\dfrac {y}{{\sqrt {x^{2}+y^{2}}}+x}}&(x>0\,\lor \,y\neq 0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}} ただし、この表示は、計算の精度が上記より下がる。
この表示は、x < 0, y = 00 / 0 浮動小数点 の計算において、計算が不安定となり、オーバーフロー する可能性がある。この範囲でのオーバーフローを避けるには、もう1つの正接の半角公式 tan θ / 2 1 − cos θ / sin θ を用いて次の計算式が使われる:
Arg ( x + i y ) = { 2 tan − 1 x 2 + y 2 − x y ( y ≠ 0 ) 0 ( x > 0 ∧ y = 0 ) π ( x < 0 ∧ y = 0 ) indeterminate ( x = y = 0 ) {\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}2\tan ^{-1}{\dfrac {{\sqrt {x^{2}+y^{2}}}-x}{y}}&(y\neq 0)\\[0.1em]0&(x>0\,\land \,y=0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}} 主値 Arg は、プログラミング言語の数学ライブラリでは関数 atan2 atan2(y , x ) の主値は区間 (−π , π ] である。
2つの複素数の乗除は、極形式表示することにより、簡明に行うことができる。複素数 z 1 , z 2
z 1 = r 1 (cos φ 1 + i sin φ 1 )z 2 = r 2 (cos φ 2 + i sin φ 2 )とすると、
arg z 1 z 2 ≡ arg z 1 + arg z 2 arg z 1 / z 2 z 1 − arg z 2 (何れも mod 2π ) z ≠ 0n が整数のとき、
arg z n n arg z (mod 2π ) 例 arg ( 2 + i ) + arg ( 3 + i ) = arg ( 2 + i ) ( 3 + i ) = arg ( 5 + 5 i ) = π 4 ( mod 2 π ) / / {\displaystyle {\begin{aligned}\arg(2+i)+\arg(3+i)&=\arg(2+i)(3+i)\\&=\arg(5+5i)\\&={\dfrac {\pi }{4}}{\pmod {2\pi }}\quad //\end{aligned}}} ^ Dictionary of Mathematics (2002). phase . ^ Knopp, Konrad ; Bagemihl, Frederick (1996). Theory of Functions Parts I and II . Dover Publications. p. 3. ISBN 0-486-69219-1 Ahlfors, Lars (1979). Complex Analysis: An Introduction to the Theory of Analytic Functions of One Complex Variable (3rd ed.). New York;London: McGraw-Hill. ISBN 0-07-000657-1 Ponnuswamy, S. (2005). Foundations of Complex Analysis (2nd ed.). New Delhi;Mumbai: Narosa. ISBN 978-81-7319-629-4 Beardon, Alan (1979). Complex Analysis: The Argument Principle in Analysis and Topology . Chichester: Wiley. ISBN 0-471-99671-8 Borowski, Ephraim; Borwein, Jonathan (2002) [1st ed. 1989 as Dictionary of Mathematics ]. Mathematics . Collins Dictionary (2nd ed.). Glasgow: HarperCollins . ISBN 0-00-710295-X 

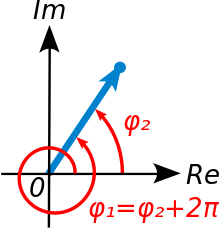
![{\displaystyle \arg z={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}&(x>0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}+\pi &(x<0\,\land \,y\geqq 0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}-\pi &(x<0\,\land \,y<0)\\[0.1em]{\dfrac {\pi }{2}}&(x=0\,\land \,y>0)\\[0.1em]-{\dfrac {\pi }{2}}&(x=0\,\land \,y<0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0f2e4f5c4b3639fa9d8154630f7192eee38a6508)
{\dfrac {\pi }{2}}&(x=0\,\land \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}+\{1-H(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0)\\[0.1em](\operatorname {sgn} y){\dfrac {\pi }{2}}&(x=0\,\land \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1b2816c91c29010b20bb66ae8311f35401aafa05)
![{\displaystyle {\begin{aligned}\arg z&={\begin{cases}|\operatorname {sgn} x|\tan ^{-1}{\dfrac {y}{x}}+{\dfrac {1-\operatorname {sgn} x}{2}}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}|\operatorname {sgn} x|\tan ^{-1}{\dfrac {y}{x}}+\{1-H_{1/2}(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/a54563b564381222765c7854d7139a2270e86ae3)
![{\displaystyle {\begin{aligned}\arg z&={\begin{cases}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\cos ^{-1}{\dfrac {x}{|z|}}&(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\{2H_{1}(y)-1\}\cos ^{-1}{\dfrac {x}{|z|}}&(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9d3f90aecd90e4901a8f2164fa67945eb11aa79c)
![{\displaystyle {\begin{aligned}\arg z&={\begin{cases}(1+\operatorname {sgn} x-|\operatorname {sgn} x|)\sin ^{-1}{\dfrac {y}{|z|}}+{\dfrac {|\operatorname {sgn} x|-\operatorname {sgn} x}{2}}(1+\operatorname {sgn} y-|\operatorname {sgn} y|)\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\\&={\begin{cases}\{2H_{1}(x)-1\}\sin ^{-1}{\dfrac {y}{|z|}}+\{1-H_{1}(x)\}\{2H_{1}(y)-1\}\pi &(x\neq 0\,\lor \,y\neq 0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/06ab2074ed145890ba666559587f92b8fe7f9ed5)






![{\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}\tan ^{-1}{\dfrac {y}{x}}&(x>0)\\[0.2em]\tan ^{-1}{\dfrac {y}{x}}+\pi &(x<0\,\land \,y\geqq 0)\\[0.1em]\tan ^{-1}{\dfrac {y}{x}}-\pi &(x<0\,\land \,y<0)\\[0.1em]{\dfrac {\pi }{2}}&(x=0\,\land \,y>0)\\[0.1em]-{\dfrac {\pi }{2}}&(x=0\,\land \,y<0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8d3fd288594d50084d430cf709ae74a35c9fa8b9)
![{\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}{\dfrac {\pi }{2}}-\tan ^{-1}{\dfrac {x}{y}}&(y>0)\\[0.1em]-{\dfrac {\pi }{2}}-\tan ^{-1}{\dfrac {x}{y}}&(y<0)\\[0.1em]0&(x>0\,\land \,y=0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/06c345d8ff813c5ea5f3a17ca85c371525d20548)
![{\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}2\tan ^{-1}{\dfrac {y}{{\sqrt {x^{2}+y^{2}}}+x}}&(x>0\,\lor \,y\neq 0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8c229242d14cc20d6a70767425f43a97261770a5)
![{\displaystyle \operatorname {Arg} (x+iy)={\begin{cases}2\tan ^{-1}{\dfrac {{\sqrt {x^{2}+y^{2}}}-x}{y}}&(y\neq 0)\\[0.1em]0&(x>0\,\land \,y=0)\\[0.1em]\pi &(x<0\,\land \,y=0)\\[0.1em]{\text{indeterminate}}&(x=y=0)\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/ee3f59db0bf5a159ddc4f0399d0c0f9205e26199)
