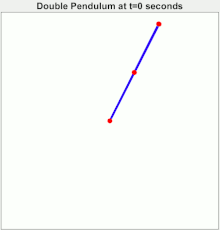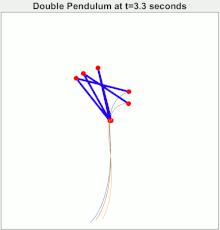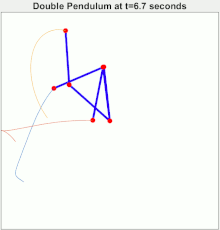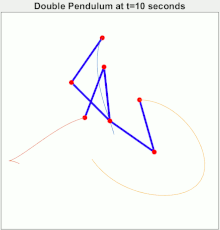
双摆

双摆是将一根单摆连接在另一个单摆的尾部所构成的系统。双摆同时拥有着简单的构造和复杂的行为。高能量双摆的摆动轨迹表现出对于初始状态的极端敏感。两个初始状态差异极小的双摆在一段时间的运行后表现非常不同，是一种具有混沌性质的简单动力系统^[1][2]。

可以考慮許多不同種類的双摆：二個擺的長度及重量可能相同，也可能不同。二個擺可能都是單擺，也有可能是複擺（compound pendulum），其運動可能限制在二維空間，也可以在三維空間內進行。在以下的分析中，二個擺的擺長l及質量都相同m，運動限制在二維空間內。

複擺的質量假設是延著其長度均勻分佈，則其複擺的質心是在中點，複擺的臂對中點的轉動慣量為I = 1/12ml²。

比較方便定義系統位形空间的方式是用複擺臂和垂直線之間的夾角為廣義座標。角度名稱為θ₁及θ₂。二桿質心的位置可以用二個座標表示。若笛卡尔坐标系的原點是在第一個擺（最上方擺）的固定點，則其第一個擺的質心在：

${\displaystyle {\begin{aligned}x_{1}&={\frac {l}{2}}\sin \theta _{1}\\y_{1}&=-{\frac {l}{2}}\cos \theta _{1}\end{aligned}}}$

第二個擺的質心在：

${\displaystyle {\begin{aligned}x_{2}&=l\left(\sin \theta _{1}+{\tfrac {1}{2}}\sin \theta _{2}\right)\\y_{2}&=-l\left(\cos \theta _{1}+{\tfrac {1}{2}}\cos \theta _{2}\right)\end{aligned}}}$

上述資訊已經可以建立拉格朗日量（Lagrangian）。

双摆系統的拉格朗日量為

${\displaystyle {\begin{aligned}L&={\text{kinetic energy}}-{\text{potential energy}}\\&={\tfrac {1}{2}}m\left(v_{1}^{2}+v_{2}^{2}\right)+{\tfrac {1}{2}}I\left({{\dot {\theta }}_{1}}^{2}+{{\dot {\theta }}_{2}}^{2}\right)-mg\left(y_{1}+y_{2}\right)\\&={\tfrac {1}{2}}m\left({{\dot {x}}_{1}}^{2}+{{\dot {y}}_{1}}^{2}+{{\dot {x}}_{2}}^{2}+{{\dot {y}}_{2}}^{2}\right)+{\tfrac {1}{2}}I\left({{\dot {\theta }}_{1}}^{2}+{{\dot {\theta }}_{2}}^{2}\right)-mg\left(y_{1}+y_{2}\right)\end{aligned}}}$

第一項是質心的平移动能，第二項是擺延著質心旋轉的轉動動能，最後一項是双摆在均勻重心場下的势能。其點標示表示變數的时间导数。

將以上的座標代入，重組後可得

${\displaystyle L={\tfrac {1}{6}}ml^{2}\left({{\dot {\theta }}_{2}}^{2}+4{{\dot {\theta }}_{1}}^{2}+3{{\dot {\theta }}_{1}}{{\dot {\theta }}_{2}}\cos(\theta _{1}-\theta _{2})\right)+{\tfrac {1}{2}}mgl\left(3\cos \theta _{1}+\cos \theta _{2}\right).}$

這裡只有一個守恆量（能量），沒有守恆的動量，二個廣義的動量可以表示為

${\displaystyle {\begin{aligned}p_{\theta _{1}}&={\frac {\partial L}{\partial {{\dot {\theta }}_{1}}}}={\tfrac {1}{6}}ml^{2}\left(8{{\dot {\theta }}_{1}}+3{{\dot {\theta }}_{2}}\cos(\theta _{1}-\theta _{2})\right)\\p_{\theta _{2}}&={\frac {\partial L}{\partial {{\dot {\theta }}_{2}}}}={\tfrac {1}{6}}ml^{2}\left(2{{\dot {\theta }}_{2}}+3{{\dot {\theta }}_{1}}\cos(\theta _{1}-\theta _{2})\right).\end{aligned}}}$

上式可以求得

${\displaystyle {\begin{aligned}{{\dot {\theta }}_{1}}&={\frac {6}{ml^{2}}}{\frac {2p_{\theta _{1}}-3\cos(\theta _{1}-\theta _{2})p_{\theta _{2}}}{16-9\cos ^{2}(\theta _{1}-\theta _{2})}}\\{{\dot {\theta }}_{2}}&={\frac {6}{ml^{2}}}{\frac {8p_{\theta _{2}}-3\cos(\theta _{1}-\theta _{2})p_{\theta _{1}}}{16-9\cos ^{2}(\theta _{1}-\theta _{2})}}.\end{aligned}}}$

運動方程式為

${\displaystyle {\begin{aligned}{{\dot {p}}_{\theta _{1}}}&={\frac {\partial L}{\partial \theta _{1}}}=-{\tfrac {1}{2}}ml^{2}\left({{\dot {\theta }}_{1}}{{\dot {\theta }}_{2}}\sin(\theta _{1}-\theta _{2})+3{\frac {g}{l}}\sin \theta _{1}\right)\\{{\dot {p}}_{\theta _{2}}}&={\frac {\partial L}{\partial \theta _{2}}}=-{\tfrac {1}{2}}ml^{2}\left(-{{\dot {\theta }}_{1}}{{\dot {\theta }}_{2}}\sin(\theta _{1}-\theta _{2})+{\frac {g}{l}}\sin \theta _{2}\right).\end{aligned}}}$

最後四個方程是有系統目前狀態時，系統隨時間演進的顯式方程。不太可能再進一步求得方程的積分解析解，得到θ₁和θ₂時間顯函數的解。不過利用龙格－库塔法或其他數值方式，可以進行數值積分來求解。

双摆的運動是混沌運動，且對初始條件非常敏感。右圖是雙擺在不同初始條件下，是否會翻倒（成為倒擺）的圖。其θ₁初始值的範圍是在x方向的−3到3，而θ₂初始值的範圍是在y方向的−3到3。點的顏色說明擺在以下時間內會翻倒：

• 10√l⁄g（綠色）
• 100√l⁄g（紅色）
• 1000√l⁄g（紫色）
• 10000√l⁄g（蓝色）

若在10000√l⁄g時間後，仍然不會翻倒，其顏色為白色。

中心白色區域的邊界可以依能量守恆推得，為以下的曲線：

${\displaystyle 3\cos \theta _{1}+\cos \theta _{2}=2.}$

因此若

${\displaystyle 3\cos \theta _{1}+\cos \theta _{2}>2,}$

以能量的關係，双摆不可能翻倒。在此區域外，以能量來說，双摆有可能翻倒，但是否會翻倒本身是很複雜的問題。若雙擺的末端是點質量，不是質量均勻分佈的桿子，情形類似^[3]。

雙擺沒有自然共振頻率，因此可用在大樓抗震設計的雙擺系統（英语：Tuned mass damper）中，大樓本身是主要的倒擺，而上面又有一個質量，形成倒雙擺。

• 单摆
• 倒雙擺
• 布萊克本擺（英语：Blackburn pendulum）

• Meirovitch, Leonard. Elements of Vibration Analysis 2nd. McGraw-Hill Science/Engineering/Math. 1986. ISBN 0-07-041342-8. 
• Eric W. Weisstein, Double pendulum （页面存档备份，存于互联网档案馆） (2005), ScienceWorld (contains details of the complicated equations involved) and "Double Pendulum （页面存档备份，存于互联网档案馆）" by Rob Morris, Wolfram Demonstrations Project, 2007 (animations of those equations).
• Peter Lynch, Double Pendulum, (2001). (Java applet simulation.)
• Northwestern University, Double Pendulum （页面存档备份，存于互联网档案馆）, (Java applet simulation.)
• Theoretical High-Energy Astrophysics Group at UBC, Double pendulum, (2005).

• 麻省理工学院TSG小组进行的双摆行为演示（实物）（页面存档备份，存于互联网档案馆）
• Youtube上的双摆轨迹演示（电脑模拟）（页面存档备份，存于互联网档案馆）